Joachim Kuczynski, 24. Oktober 2023
Einleitung
In diesem Artikel möchte ich die Abhängigkeit des Optionswerts von der Eintrittswahrscheinlichkeit der realen (binomialen) Zustände innerhalb des Modells von Cox, Ross and Rubinstein darstellen. Auch in der Literatur ist mancherorts zu lesen, dass die risikoneutralen Wahrscheinlichkeiten und damit die Optionswerte nicht von den Wahrscheinlichkeiten der Realzustände abhängen. Die Optionswerte hängen aber implizit sehr wohl von ihnen ab. Dies möchte ich in diesem Post kurz darstellen und herleiten.
Binomialmodel von Cox, Ross und Rubinstein
Optionen können mit dem Binomialmodell von Ross, Cox and Rubinstein bewertet werden. Der Optionswert 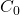 zum Zeitpunkt
zum Zeitpunkt 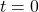 ist:
ist:
![Rendered by QuickLaTeX.com \[C_0=\frac{\alpha C_{u,t_1}+(1-\alpha )C_{d,t_1}}{(1+r)^T }\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-d245e3e501c03334a52b0327fc44aea3_l3.png)
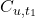 and
and 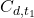 sind die Optionswerte der up und down Entwicklungen zum Zeitpunkt
sind die Optionswerte der up und down Entwicklungen zum Zeitpunkt 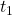 .
.  ist der risikofreie (bzw. zuschlagsfreie) Zinssatz und T die Zeit zwischen
ist der risikofreie (bzw. zuschlagsfreie) Zinssatz und T die Zeit zwischen  und
und 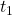 .
. 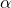 ist hierin die risikoneutrale Wahrscheinlichkeit der up Bewegung in
ist hierin die risikoneutrale Wahrscheinlichkeit der up Bewegung in 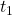 ,
, 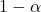 ist die risikoneutrale Wahrscheinlichkeit der down Bewegung in
ist die risikoneutrale Wahrscheinlichkeit der down Bewegung in 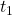 . Das Binomialmodel stellt uns folgende Beziehung zur Verfügung:
. Das Binomialmodel stellt uns folgende Beziehung zur Verfügung:
![Rendered by QuickLaTeX.com \[\alpha=\frac{(1+r)^T-d}{u-d}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-53e84d5ecdafd962f48030489a62864d_l3.png)
Einsetzen von 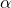 liefert uns diese Darstellung für
liefert uns diese Darstellung für 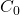 :
:
![Rendered by QuickLaTeX.com \[C_0=\frac{\frac{(1+r)^T-d}{u-d} C_{u,t_1}+(1-\frac{(1+r)^T-d}{u-d} )C_{d,t_1}}{(1+r)^T }\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-4585ecf3307b3ea36a992a134bd156a8_l3.png)
Nach einer kleinen Umformung erhalten wir:
![Rendered by QuickLaTeX.com \[C_0=\frac{( (1+r)^T-d ) C_{u,t_1}+(u-(1+r)^T )C_{d,t_1}}{(1+r)^T (u-d)}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-e41bd7434143afaf1fc36677db98383c_l3.png)
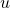 und
und  sind definiert als die Verhältnisse von up und down Entwicklung zum Erwartungswert des Zustands in
sind definiert als die Verhältnisse von up und down Entwicklung zum Erwartungswert des Zustands in  ,
, 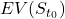 :
:
![Rendered by QuickLaTeX.com \[u= \frac{EV(S_{t_0})}{S_{u,t_1}}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-d9deab9057d9c6d1f3a1ba8f0e6ce779_l3.png)
![Rendered by QuickLaTeX.com \[d= \frac{EV(S_{t_0})}{S_{d,t_1}}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-875c14eb30bf1a7d78b5173c9c6dfadf_l3.png)
Bis jetzt sind die Wahrscheinlichkeiten von Zustand up 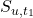 und Zustand down
und Zustand down 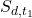 nicht vorgekommen. Vielfach wird nun argumentiert, dass die Wahrscheinlichkeiten der beiden Realzustände den Optionswert nicht beeinflussen. Dies stimmt nicht. Der Erwartungwert von Zustand
nicht vorgekommen. Vielfach wird nun argumentiert, dass die Wahrscheinlichkeiten der beiden Realzustände den Optionswert nicht beeinflussen. Dies stimmt nicht. Der Erwartungwert von Zustand 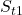 und damit von
und damit von  hängen von diesen Wahrscheinlichkeiten ab. Der Erwartungswert des Zustands in
hängen von diesen Wahrscheinlichkeiten ab. Der Erwartungswert des Zustands in  ist der diskontierte Wert des Zustands in
ist der diskontierte Wert des Zustands in 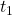 . Mit
. Mit 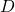 als jährlichen kostanten Diskontierungsrate erhalten wir:
als jährlichen kostanten Diskontierungsrate erhalten wir:
![Rendered by QuickLaTeX.com \[EV(S_{t_0})=\frac{EV(S_{t_1})}{(1+D)^T}=\frac{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}{(1+D)^T}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-1bde57d477d9f69bdf954feb24df97ba_l3.png)
Für 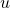 und
und  erhalten wir:
erhalten wir:
![Rendered by QuickLaTeX.com \[u=\frac{EV(S_{t_0})}{S_{u,t_1}}=\frac{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}{S_{u,t_1}(1+D)^{T}}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-ba3a6fc511e4ae945efcc80c544226d2_l3.png)
![Rendered by QuickLaTeX.com \[d=\frac{EV(S_{t_0})}{S_{u,t_1}}=\frac{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}{S_{d,t_1}(1+D)^{T}}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-df6488617bbde3eaabec1cf22b39708f_l3.png)
Das Endergebnis lautet nun:
![Rendered by QuickLaTeX.com \[C_0=\frac{( (1+r)^T-\frac{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}{S_{d,t_1}(1+D)^{T}}) C_{u,t_1}}{(1+r)^T ( \frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{d,t_1}(1+D)^{T}}})}+\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-0769b16c961ea4a991747ee6dec7ad23_l3.png)
![Rendered by QuickLaTeX.com \[+\frac{(\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-(1+r)^T )C_{d,t_1}}{(1+r)^T ( \frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{d,t_1}(1+D)^{T}}})}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-5b78a3049855170fe758d7f83b5e5355_l3.png)
Dies ist die grundlegende Beziehung zwischen Optionswert zum Zeitpunkt  und expliziten problemspezifischen Variablen.
und expliziten problemspezifischen Variablen.
Ergebnis
Wir sehen, dass der Optionswert 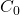 explizit von den Wahrscheinlichkeiten
explizit von den Wahrscheinlichkeiten 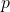 und
und  der realen up
der realen up  und down Zustände
und down Zustände 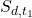 abhängt. Das wollten wir zeigen. q.e.d.
abhängt. Das wollten wir zeigen. q.e.d.
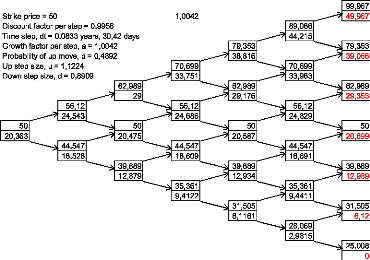
![Rendered by QuickLaTeX.com \[C_0=\frac{\frac{(1+r)^T-d}{u-d} C_{u,t_1}+(1-\frac{(1+r)^T-d}{u-d} )C_{d,t_1}}{(1+r)^T }\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-4585ecf3307b3ea36a992a134bd156a8_l3.png)
![Rendered by QuickLaTeX.com \[C_0=\frac{( (1+r)^T-\frac{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}{S_{d,t_1}(1+D)^{T}}) C_{u,t_1}}{(1+r)^T ( \frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{d,t_1}(1+D)^{T}}})}+\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-0769b16c961ea4a991747ee6dec7ad23_l3.png)
![Rendered by QuickLaTeX.com \[+\frac{(\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-(1+r)^T )C_{d,t_1}}{(1+r)^T ( \frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{u,t_1}(1+D)^{T}}}-\frac{{pS_{u,{t_1}}+(1-p)S_{d,{t_1}}}}{{S_{d,t_1}(1+D)^{T}}})}\]](https://www.financeinvest.at/wp-content/ql-cache/quicklatex.com-5b78a3049855170fe758d7f83b5e5355_l3.png)