DCF Analysis - Investment and Project Valuation

Operating Leverage in Practice

Joachim Kuczynski, 15 December 2023

Introduction

A key issue in each asset valuation is the calculation of an asset’s equity beta. The preferred procedure is to take the appropriate industry segment equity beta and adjust it to your specific asset. This adjustment mainly consists of financial leverage and operating leverage. In this article I explain the procedure of operating leverage adjustment. In the following discussion we name the asset, that we want to value, our project. In general, the operating leverage of all assets can be analyzed this way. Especially it is also valid for single contribution cash flows, if you want to use the approach in the component cash flow procedure, which I am preferring in my valuations because of its accuracy.

Unleveraging

Most valuations base on average industry segment equity betas, which are adapted to the specific asset. We receive equity industry segment betas 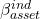$\beta^{ind}_{asset}$ from databases in literature or in the web. In my articles concerning operating leverage and operating leverage in CCFP you can see, that the average industry equity beta for revenues and variable costs can be calculated in this way:

$\beta^{ind}_{rev}=\beta ^{ind}_{asset} \left( 1-\frac{V^{ind}_{fix}}{V^{ind}_{asset}} \right)^{-1}$

The equity beta for revenues and variable costs are the same for the industry segment and for the considered project. That means 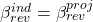$\beta^{ind}_{rev} = \beta^{proj}_{rev}$ . Hence we obtain:

$\beta^{proj}_{rev}=\beta ^{ind}_{asset} \left( 1-\frac{V^{ind}_{fix}}{V^{ind}_{asset}} \right)^{-1}$

This is an important result for the component cash flow procedure. It provides the equity beta for revenues and variable cost cash flows. Using the CAPM you derive the discount rates for revenues and variable cost cash flows.

Releveraging

We can calculate the asset equity beta of the project as well:

$\beta^{proj}_{asset}=\beta ^{proj}_{rev} \left( 1-\frac{V^{proj}_{fix}}{V^{proj}_{asset}} \right)=$

$= \beta ^{ind}_{asset} \frac{1-\frac{V^{proj}_{fix}}{V^{proj}_{asset}}}{1-\frac{V^{ind}_{fix}}{V^{ind}_{asset}}}$

This is the appropriate equity beta of the considered project, if you use the project cash flow procedure. Making use of the CAPM you get the discount rates for the project’s cash flows in general with the corrected operating leverage.

Example

We want to value a simple project with infinite lifetime, a turnover of EUR 120 and costs of EUR 50 per year. The yearly profit is EUR 70. The riskless rate is 5%, the expected market return rate is 10%.

Case 1: The project has only variable costs and no fixed costs. The project has a beta of 1. The discount rate of the project (CAPM) is r=5%+1.0 (10%-5%)=10%. With infinite project lifetime we get a project value of EUR 70 / 10% = EUR 700.

Case 2: Let us assume now the EUR 50 cost per year to be fixed instead of variable. At first we discount the cash flows seperately acc. to the component cash flow procedure. Turnover still has a beta of 1 and a discount rate of 10%. We obtain a turnover value of EUR 120 / 10% = EUR 1200. The fixed costs have to be discounted with the riskless rate of 5%. Hence we get a value of EUR 50 / 5% = EUR 1000. The project value is EUR 1200 – EUR 1000 = EUR 200.
Next we apply the project cash flow procedure, which discounts all cash flows with a unique project discount rate. The beta of the project’s revenues does not depend on the fixed costs share, it is still 1. With formula described above we can calculate the project beta with the new operating leverage:

$\beta^{project}_{asset}=1.0 \left( 1+ \frac {1000}{200} \right)=6$

A second way to calculate the project beta is by taking the weighted average of its components’ betas:

$\beta_{P}=\frac{R}{R-C}\beta_{R} - \frac{C}{R-C}\beta_{C}=$

$=\frac{1200}{1200-1000}1 - \frac{1000}{1200-1000}0=6$

Both approaches provide a project beta of 6. According to CAPM we get a project discout rate of r=5%+6(10%-5%)=35%. Hence we obtain a project value of EUR 70 / 35% = EUR 200, the same result as calculated with the component cash flow procedure.

But the key message of this example is that the value of the project falls from EUR 700 to EUR 200 because of higher operating leverage. This illustrated that an adaption of betas and discount rates because of operating leverage is crucial in many cases. Only in this way we can get valid results.

In more complex projects with many different kind of cash flows I prefer the component cash flow analysis. A change in one cash flow changes only the value of this cash flow. If you use the project cash flow procedure, you always have to calculate a new project discount rate at any change of one parameter.

Approximation with P&L statement

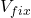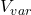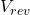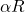The (present) values of fixed costs $V_{fix}$ , variable costs $V_{var}$ and revenues $V_{rev}$ cannot be found easily. But we can try to approximate them with P&L or balance sheet figures, which are available more eaysily. Let us consider eternal yearly revenues $R$ , yearly fixed costs $\alpha R$ and yearly variable costs 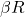$\beta R$ . The risk free rate is $r_f$ and the market return rate $r_m$ . 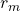With the expressions above we get the beta of the asset:

$\beta _{asset} = \beta_{rev} \left( 1 - \frac{V_{fix}}{V_{asset}} \right)$

The values of fixed costs and asset are:

$V_{fix}=- \frac{\alpha R}{r_f}$

$V_{asset}= \frac{R - \alpha R - \beta R}{r_m}$

With that we obtain for 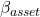$\beta_{asset}$ :

$\beta _{asset} = \beta_{rev} \left( 1 + \frac{\frac{\alpha R}{r_f}}{\frac{R - \alpha R - \beta R}{r_m}} \right) = \beta_{rev} \left( 1 + \frac{r_m}{r_f}\frac{\alpha}{1-\alpha-\beta} \right)$

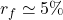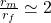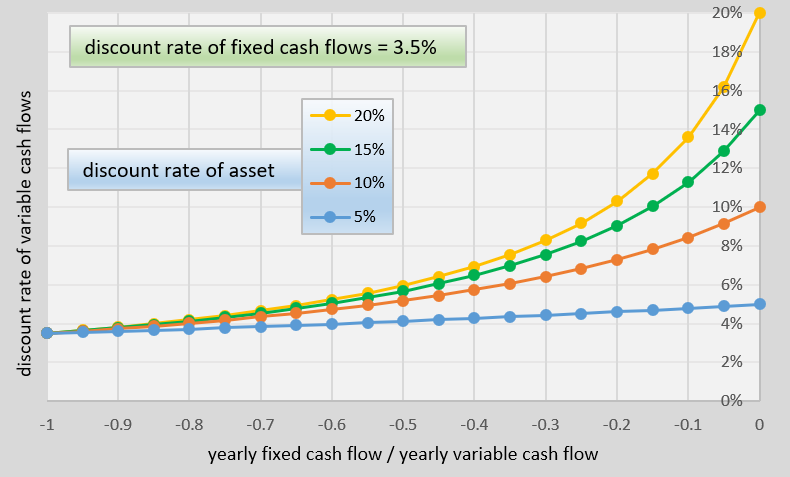
$r_m \simeq 10 \%$ and $r_f \simeq 5 \%$ approximately, so 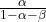$\frac{r_m}{r_f} \simeq 2$ , $\frac{\alpha}{1-\alpha-\beta}$ is the ratio of annual fixed costs to annual profit. That means that you have to multiply that factor with the 2, when applying P&L figures in approximation and not present values.

Lease or Buy / Make

Joachim Kuczynski, 03 April 2023

A rental agreement that extends for a year or more by a series of fixed payments is called a lease. Firms lease as an alternative to buying capital equipment. Cars, aircraft, ships, farm equipment and trucks are leased many times. In principal every kind of asset can be leased. In this post I want to describe the valuation of a lease contract and how you can analyse whether to prefer buying / making or leasing an asset from the financial point of view.

The correct way

At first you have to figure out all free cash flows that are different between buying an asset in comparison to leasing it. Usually this concerns cash flows from purchasing, tax shield because of EBIT reduction caused by depreciation or leasing payments, tax shields because of debt interest deduction, maintenance costs and salvage value of the asset. After that you have to discount all cash flows with the appropriate risk adjusted discount factor ( $\gamma_{b,i}$ for the buying and $\gamma_{l,j}$ for the leasing scenario). Adding up all present values you get a net present value (NPV) of the buying case and a NPV of the leasing case.

$NPV_b=\sum_{i}^{}\gamma_{b,i}C_{b,i}$

$NPV_l=\sum_{j}^{}\gamma_{l,j}C_{l,j}$

The case with the higher NPV is the better one from financial point of view. That means leasing is better than buying, if $NPV_l > NPV_b$ .

Many firms do not value this way because they are not familiar with risk appropriate discounting of cash flows. Therefore they use simplified formulas described in many corporate finance books. But in general that can lead to false results if the premises of the simplifications do not fit reality. So take care and do not use the simplified formulas without checking the concrete situation.

Simplified ways

The short ways are characterized by unifying discount factors for cash flows. This assumes that the cash flows have the same risk adjustment. In general that is not the case obviously. Each cash flow has its own risk in principle. In my calculation I consider each cash flow and calculate its specific risk. That is not much additional work. But I can be sure that I get the correct result.

In some cases you can assume the same discount factor. Tax shield because of EBIT reduction, caused by depreciation and leasing payments, and debt interest deduction can be considered as fixed cash flows in most cases. They have no market dependency. Hence you can discount their cash flows with the company’s debt interest rate. Depending on the debt interest deductibility of the cash flows you have to take the before or after interest tax rate (operating and financial lease).

If some cash flows have market dependency (e.g. salvage value) or are realised in different currencies, the discount factors might not be the same. Additionally, the changing capital structure and the dependency of tax deductibility on the market development can lead to different discount rates. In all these cases you cannot use the simplifies formulas. Then you have to apply the APV method and calculate all NPV contributions seperately. This is the procedure of the previous section.

Debt-equivalent cash flows

The authors of many books about corporate finance use the term debt-equivalent cash flows. That are the additional cash flows that occur when financing a free cash flow stream by an equivalent loan. At leasing vs buying the free cash flow stream is leasing FCF minus buying FCF. After that you calculate interests of the loan and the interest tax shields with APV or with simplified adjusted discount rates (as described before). But take care! This simplified version is only valid, if leasing and buying cash flows have the same discount factor (risks) in each period. Otherwise the calculation with debt-equivalent cash flows provides false results!

Options in leasing contracts

Many leasing contracts include options like buying the assets at the end of the leasing time or cancelling the leasing contract before expiration. Any option can be valued with Real Options Analysis. The analysis is problem-specific. But in general each option in a leasing contract has a specific, well defined value.

Conclusion

The analysis of leasing contracts (and their comparion to buying / making) is completely the standard analysis of asset valuation. But you should do that in the basic accurate way. That means DCF analysis with appropriate risk-adjusted discount rates (Component Cash Flow Procedure) including the adjusted present value (APV) approach. It is not much additional work to do. But you can be sure, that your results are right.

Return Rate Aggregation

Joachim Kuczynski, 09 February 2023

In many books you can read that the return rate of a set of several assets is the weighted average of the single asset’s return rates. But up to now I did not found any proof for this statement. In this post I provide a derivation of that relationship. An additional benefit of that calculation is to understand the conditions under which that relationship is valid basically.

Let us start with an asset value at time $t$ , $C(t)$ , which is the sum of different assets values $C_i(t)$ :

$C(t)=\sum_{i}^{}C_i(t)$

At time $t=0$ the asset values are $C(0)$ and $C_i(0)$ with $C(0)=\sum_{i}^{}C_i(0)$ . The asset value $C_i$ is developing in time $t$ with its specific return rate $r_i$ , that means:

$C_i (t)=C_i(0)exp(r_i t)$

Now we are searching an aggregated return rate $r$ , that describes the development of the aggregated asset value $C$ . Setting $C(t)=C(0)exp(rt)$ we obtain:

$r=\frac{1}{t}ln\frac{C(t)}{C(0)}=\frac{1}{t}ln\left( \sum_{i}^{} \left \frac{C_i(0)}{C(0)} exp \left( r_i t \right) \right \right)$

This is the exact relationship between the aggregated return rate $r$ and the differential return rates $r_i$ . This expression cannot be simplified any more. Now we develop the exponential and logarithmic functions using Taylor series and take the polynomial approximation only up to its first oder. That means $\text{exp}\left( x \right)\simeq 1+x$ and $\text{ln}\left( x \right)\simeq x-1$ . Hence we get a first order approximation of $r$ :

$r\simeq \frac{1}{t}\left( \sum_{i}^{} \left( \frac{C_i(0)}{C(0)} \left( 1+ r_i t \right) \right) -1 \right)$

This simplyfies to:

$r\simeq \sum_{i}^{} \frac{C_i(0)}{C(0)} r_i$

This is the result, that many authors present and use in their books. Also the calculation of the WACC, or aggregated return / discount rate respectively, is told to be the weighted average of debt $D$ return rate $r_D$ and equity $E$ return rate $r_E$ :

$WACC=r=\frac{D}{E+D}r_D+\frac{E}{E+D}r_E$

But take care, that all is only an approximation. And in some cases is can be an inaccurate approximation. With increasing differential return rates $r_i$ and increasing time $t$ the approximation becomes more and more inaccurate. If you require an exact calculation, take the formula presented above.

Note that it does not matter whether you take $C_i (t)=C_i(0)exp(r_i t)$ or $C_i (t)=C_i(0)(1+r_i^*)^t$ . With a substitution of $r=ln(1+r^*)$ , you can transform these two return rates into each other.

Operating Leverage in CCFP

Joachim Kuczynski, 04 February 2023

Introduction

The only correct way to discount cash flows and value an asset is the Component Cash Flow Procedure (CCFP). Hereby each cash flow is discounted with its appropriate risk-adjusted discount rate. All fixed cash flows, that have no market risk and are diversifiable for investors, have to be discounted without additional equity risk premiums. Fixed cash flows include fixed operative costs, R&D, investments and cash flows for fixed expenditures like customer and tooling expenses & payments. In contrast to that contribution cash flows depend on the market development. They must be discounted with rates that include equity risk premiums for the investors. Primarily, contribution cash flows consist of turnover, variable costs and working capital cash flows.

In most cases discount rates for contribution cash flow are based on bottom-up betas and not on historical data. These are averaged equity betas representing an average of similar companys in an industry segment in a certain country or market. But these bottom-up betas take into account all cash flows, contribution cash flows as well as fixed cash flows. Hence these bottom-up betas are not correct to discount contribution cash flows alone. But that is exactly what is required in the CCFP. The content of this post is the derivation of the contribution cash flow beta from the bottom-up industry segment beta.

Derivation

Base for the derivation is the market balance sheet where the value of the asset (project or investment), $V_{asset}$ , is the sum of revenues’ value, $V_{rev}$ , the value of variable cost cash flows, $V_{var}$ , and value of the fixed cost cash flows, $V_{fix}$ :

$V_{asset}=V_{rev}+V_{var}+V_{fix}$

Now we derive in respect to the market portfolio return rate $r_m$ and devide by $V_{asset}$ . The relative change of $V_{asset}$ in respect to $r_m$ is just the beta of the asset, $\beta_{asset}$ , by definition. Hence we get

$\beta_{asset}=\frac{\frac{\partial }{\partial r_m}V_{asset}}{V_{asset}}=\frac{\frac{\partial }{\partial r_m} V_{rev} + \frac{\partial }{\partial r_m} V_{var}+ \frac{\partial }{\partial r_m} V_{fix}}{V_{asset}}.$

The fixed cash flows are independent of the market return rate. That means that the derivation $\frac{\partial }{\partial r_m} V_{fix}=0$ and we get:

$\beta_{asset}=\frac{\frac{\partial }{\partial r_m} V_{rev} + \frac{\partial }{\partial r_m} V_{var}}{V_{asset}}=\frac{V_{rev}\beta_{rev} + V_{var}\beta_{var}}{V_{asset}}$

The betas of revenues and variable costs are the same, $\beta_{rev}=\beta_{var}$ . We get:

$\beta_{asset}=\beta_{rev} \frac{V_{rev} + V_{var}}{V_{asset}}=\beta_{rev} \left( 1-\frac{V_{fix}}{V_{asset}} \right)$

Note that the value of the fixed cash flows is negative, $V_{fix} \le 0$ , and the value of the asset is positive, $V_{asset} \ge 0$ , in ordinary cases. The expression in the bracket becomes $\ge 1$ . That means that the beta of the asset is higher than the beta of the revenues, $\beta_{asset} \ge \beta_{rev}$ . Obviously that is true, because adding fixed costs increases the beta of the asset. This effect is known as operating leverage.

Application in CCFP

The bottom up beta from an industry segment includes fixed costs, it corresponds to $\beta_{asset}$ . Applying the previous derivation you can calculate the beta of the contribution cash flows, $\beta_{rev}$ and $\beta_{var}$ . $V_{fix}$ and $V_{asset}$ are values from the market balance sheet of the averaged industry segment companies. If these values are more or less stable over time you can also take the corresponding figures from the averaged income statement of the industry segment. These data are available in several statistical sources. With $\beta_{rev}$ you can calculate the equity risk premium of the contribution cash flow discount rate and hence the discount rate itself, for instance according to the capital asset pricing model (CAPM). With $r_f$ as risk free rate and $r_m$ as average return rate of the industry segment, the equity return rate is:

$r_E=r_f+\beta _{rev}\left( r_m - r_f \right)$

The return rate for contribution cash flows $r_{rev}$ considering debt $D$ and equity $E$ financing including tax shield effect with constant debt to equity ratio and marginal tax rate $t$ is:

$r_{rev}=\frac{D}{E+D} \left( 1-t \right) r_D + \frac{E}{E+D} r_E$

With that procedure we get the correct discount rate for the contribution cash flows in the CCFP approach.

If there are several contribution cash flows from different industry segments with specific risks, we can do that procedure with each kind of contribution cash flow. In this way we get the appropriate return rate for each kind of contribution cash flow.

Profitability Index Annuity

Joachim Kuczynski, 29 September 2022

Project ranking is an important issue in capital rationing, when the company has limited financial resources. A company can rank its projects with various measures: NPV, eNPV, PI, PIA, IRR, MIRR, Baldwin rate of return and many others. The profitability index annuity is a further development of the profitability index to consider the temporal cash flow distribution in the decision making process.

Profitability Index

The profitability index (PI) of an investment project is the present value of all cash flows without investment (let us name them contribution cash flows), $PV_C$ , divided by the present value of all investment cash flows, $PV_I$ . Hereby it is important how we define the term investment. You can have a look at my proposal in this post. Hence we get for the profitibility index:

$PI=\frac{PV_C}{PV_I}$

The $PI$ shows how much value is created per investment. All projects with a $PI>1$ are creating additional value to the investors, all projects with a $PI<1$ are destroying value.

Sometimes the profitability index is defined net present value of the project devided by the present value of all investment cash flows. Let us name this definition of the profitability index PI*:

$PI^*=\frac{PV_C-PV_I}{PV_I}=\frac{PV_C}{PV_I}-1=PI-1$

No matter which definition we take, the ranking of projects remains the same. The difference is only an “offset” of 1.

Profitability Index Annuity

Most managers prefer projects in which the returns of the investment are in the beginning. They prefer early cash flow return. The profitability index cannot provide any information concerning the timing of the cash flows. The profitability index annuity (PIA) tries to solve that issue. It is defined as profitability index divided by the annuity factor $A_{n,r}$ :

$PIA=\frac{PI}{A_{n,r}}$

An annuity is a sequence of equal cash flows paid each period for a specified number of periods $n$ . The sum of all the discount factors equals the annuity factor. With $n$ as the project lifetime and $r$ as annual discount rate, the annuity factor is defined as:

$A_{n,r}=\frac{1}{r}\left( 1-\frac{1}{\left( 1+r \right)^n} \right)$

The previous equation is only valid, if all cash flows are discounted with the same discount rate in all periods. If you have different discount rates in the considered periods, the formula not valid any more but can be adapted easily. If you use the Component Cash Flow Procedure, there might be no solution or more than one possible solution for the PIA value. So take care when applying the PIA ranking!

Conclusion

The annuity factor considers how much the investment project is influenced by the discounting effect. An investor that prefers early cash flow returns wants to have small discounting effects. The smaller the discounting effect the smaller the annuity factor and the higher the profitability index annuity. Looking at two projects with the same PI the investor prefers the project with the higher PIA.

Project rankings based on the profitability index annuity (PIA) have some desirable properties. Projects with shorter lives tend to have higher ranking PIAs, and short life implies rapid cash generation. Choosing projects ranked by their PIA values can help managers to invest in projects combining three desirable characteristics: High PV, low capital requirement and rapid cash generation.

In my point of view PIA is an additional useful figure to evaluate investments and projects. But we should not use it as the only measure. It relies on the term investment that we have to consider carefully. Always think about what is the true financial limitation in the company and then refer to that.

Investment Term

In this post I would like to make some comments concerning the term INVESTMENT. It is a central notion in investment and project valuation. Many key figures are based on this term. Hence it is important that we really know what it is.

An asset valuation is always done from the fund / capital providers’ or investors’ sight. From that point of view an investment is the amount of cash that the investor has to provide to run the investment project. The cash flow between investor and investment project is the basis of the valuation. Thereby it is not important when the investment cash flow takes place. An investment cash flow does not has to be an initial cash flow in the beginning of the project. An investor can always shift the cash flows by using the capital markets. He can borrow money from a bank and pays it back later with additional interests.

From the investor’s point of view it is not important how the cash is handled in the income statement and balance sheet. The investor is only interested in cash flows concerning him / her. The notion investment does not depend on any classification in income statement and balance sheet. Further it is irrevelant whether the investment cash flow is depreciated / amortized or not.

The investment of a project are all fixed cash flows. That are all cash flows that have no dependency on the market devopment. These are for example cash flows for machines, land, buildings, product development, fixed costs for production, patents, fixed customer payments for various items and further more. It is not important whether these cash flows are incoming or outgoing. In a discounted cash flow analysis the investment cash flows must be discounted with the riskless rate that includes no risk premium. It is incorrect to discount investment cash flows with any discount rate including market risk premiums, for example the WACC. For that reason all figures valuing a project with a single rate (IRR, MIRR, …) are doubtful. They cannot value projects with cash flows having different risk and with it discount rates.

All cash flows that depend on the market development are not investment cash flows. That mainly includes turnover and variable costs. They must be discounted with the appropriate risk adjusted discount rate, because the investor requires a risk premium for taking that non diversifiable risks. Each risky cash flow has its specific own risk and requires an appropriate risk adjusted discount rate.

Preinreich Lücke Theorem

Joachim Kuczynski, 31 August 2022

The Preinreich Lücke Theorem tells us that the present value of the residual incomes is equal to the present value of the corresponding cash flows. This might be important, because it is the link of yearly reported figures like economic value added (EVA) to the value of a complete future cash flow stream. In this post I provide the proof of the theorem. Furthermore I want to discuss the premises and consequences critically.

Proof of Preinreich Lücke Theorem

The residual income is income minus capital costs. Capital means in this view all expenditures that are amortized and do not affect income directly. The residual income in period t is defined as:

$I_t^{res}:=f_t+c_t-c_{t-1}-c_{t-1}i_t$

$f_t$ is the cash flow in period $t$ , $c_t$ the fixed capital in period $t$ and $i_t$ the discount rate in period $t$ . $c_t - c_{t-1}$ is just the depreciation in period $t$ . The present value of the residual incomes is equal to the present value of the corresponding cash flows, if the difference of them is zero. The difference is:

$\sum_{t=0}^{n}(f_t-I_t^{res})\rho_t=\sum_{t=0}^{n}(c_{t-1}(1+i_t)-c_t)\rho_t$

$\rho_t$ is the discount factor in period $t$ and decreases in period $t$ by the factor $1+i_t$ . That means $\rho_t = \rho_{t-1} / (1+i_t)$ . With that we obtain:

$\sum_{t=0}^{n}(f_t-I_t^{res})\rho_t=\sum_{t=0}^{n}(c_{t-1}\rho_{t-1} - c_t \rho_t)$

Within that sum all terms in the middle cancel out. Only the first and the last term remain. Assuming that there is no fixed capital before $t=0$ we can set $c_{-1} = 0$ . And if all fixed asset is depreciated in the considered n periods, we can set $c_n=0$ . With these two premises we realize that all terms of the sum become zero.

$\sum_{t=0}^{n}(f_t-I_t^{res}) \rho_t = c_{-1} \rho_{-1} - c_n \rho_n=0$

That means that there is no difference of discounting cash flows or discounting residual incomes. This is exactly what we wanted to proof.

Discussion

At first I want to point out that the Preinreich Lücke Theorem requires the same discount factor in one period i_t for all cash flows. They can differ from one period to another, but within the same period all cash flows and residual incomes are discounted with the same factor. In reality each cash flow can have its own risks (risk premiums) and its own financing structure. That means that each cash flow can require its own specific appropriate discount factors. But with different discount factors the Preinreich Lücke Theorem does not work any more.

Secondly, the fixed capital must be amortized completely in the considered n periods. If there is a residual book value in the last period n, the Preinreich Lücke Theorem is not valid any more.

As a third point I want to mention that you get residual values after having calculated the cash flows. The calculation with residual incomes is an additional calculation loop with no real benefit.

As a last point I want to mention that figures like EVA are used widely because they can be calculated in addition to an income statement easily. But this is not the same as it is done in the Preinreich Lücke Theorem. There we have a calculation of one investment / project in many periods and not only one.

Internal Rate of Return (IRR)

The internal rate of return (IRR) is a widespread used figure to evaluate investment projects. But it is a very dangerous figure that can lead to wrong decisions easily. The figure is only valid if the investment project fulfills special conditions.

IRR Definition

I want to give you a clear derivation of the IRR definition to point out some very critical issues. The internal rate of return is defined to be the zero of a polynomial that calculates the net present value (NPV) of an investment project. The NPV is the sum of all discounted incremental cash flows to the firm after taxes, $c_i$ . The discount factors $\gamma_i$ can be different for each cach flow $c_i$ .

$NPV=\sum_{i}^{}\gamma _i c_i$

Let us assume that we can sum up the products in each period $t$ , because all cash flows one period $t$ have the same discount factor $\gamma _t$ (uniformity). Let $C_t$ be the sum of the cash flows in period $t$ . Hence we can simplify the NPV calculation by summing over all time periods instead of over all cash flows:

$NPV=\sum_{t}^{}\gamma _t C_t$

Further we assume that the discount factor $\gamma_t$ has polynomial character in $t$ (flatness). In this case we can rewrite the NPV as a polynomial:

$NPV=\sum_{t}^{}C_t\gamma ^t$

Let $i$ be the annual interest rate to discount cash flows. Setting $\gamma=\left( 1+i \right)^{-1}$ we get the well-known formula:

$NPV=\sum_{t}^{}C_t\left( 1+i \right) ^{-t}$

The internal rate of return $i^{IRR}$ is now defined to be the values of $i$ for which the NPV is zero:

$NPV=\sum_{t}^{}C_t\left( 1+i^{IRR} \right) ^{-t}=0$

I want to point out that we require two restrictive premises to get an expression for the IRR, flatness and uniformity.

Flat and uniform discount rates

To give the NPV the form of a polynomial we required two important assumptions. At first the investment project must have a uniform discount rate. That means that the discount rate has to be the same for all cash flows in one period. All cash flows are assumed to have the same equity risk premium and the same risk free rate. In almost all investment projects this assumption does not hold. Secondly, the discount rate structure must be flat. That means that you have the same discount rate for each period. The risk free rate and the equity risk premiums are assumed to be constant over time. In most investment projects this is also not true.

We can state the the premises uniformity and flatness to let the NPV get a polynomial cannot be applied in almost all investment projects. Especially in complex projects with many different cash flows, currencies and risks these assumptions are not valid.

Ambiguity

The IRR are the zero points of a polynomial. A polynomial of order $n$ can have $n$ different zeros. That means that you get $n$ different IRR in general, the solution for possible IRR can be ambiguous. If you get more than one IRR, which one is the right one? Or you can even get no solution. But even when you get no solution for the IRR, there exists a contribution of the project to the company’s value. NPV (or eNPV) can evaluate all kinds of value contributions to the firm and must be preferred.

IRR decision rule

According to the decision rule of the IRR method an investment should be realized, if the IRR is bigger than the WACC. That is only true, if the the NPV has a negative derivative at the IRR. A zero point of a $n$ -order polynomial can have a positive or negative first derivation at zero points. Accordingly you cannot say that the NPV decreases with increasing discount rate in general. That means that the IRR decision rule does not hold when the first derivative is positive.

Mutually exclusive investment projects

Firms often have to choose between several alternative ways of doing the same job or using the same facility. In other words, they need to choose between mutually exclusive projects. The IRR decision rule can be misleading in that case as well. An alternative can have a bigger IRR but a smaller NPV than another alternative at the same time. The investors of the project (who are the relevant deciders) are interested in a maximum increase of value of the firm. That is represented by the NPV. Hence comparing alternatives by their IRR can lead to false decisions.

Conclusion

My conclusion is the the IRR method with its decision rule should only be applied at very simple projects with one investment in the beginning and uniform / flat discount rates. It should not be applied as decision figure in a standardized investment valuation concept of a company. In all complex projects the NPV (or eNPV) is the much better concept. Additionally the NPV / eNPV gives the investors the information they demand, namely the contribution of the investment project to the value of the firm and hence the value of their shares.

Risk-Free Rate

Importance of risk-free rates

The risk-free rate of return $r_f$ is the theoretical return rate of an investment with no risk. The risk-free rate represents the interest an investor would expect from an absolutely risk-free investment over a specified period of time. It plays a central role in financial valuation and is the planning base for return rates of risky assets.

Prospective cost of debt (return rate requirement) can be estimated by adding default spreads to the risk-free rate. This can be done by synthetic rating of the company with its interest coverage ratio and adding a default spread according to the synthetic rating of the company. Additionally, in an emerging market the country of the occuring cash flows can generate a further country default spread added to the risk free rate.

Equity return rates $r_e$ are calculated by adding risk premiums to the risk-free rate. Considering the Capital Asset Pricing Model (CAPM) the expected value of the equity return rate is:

$E \left( r_e \right)= r_f+\beta \left( E\left( r_m \right) - r_f \right)$

$\beta$ is the sensitity of the risky cash flow return rate to the efficient market protfolio return rate $r_m$ .

Risk-free rates are also very important in real options analysis. The time values of real options are weighted by risk-neutral probabilities and discounted with the risk-free rate.

Because of all these reasons it is very important to understand the risk-free rate in detail. Most books neglect that issue. In my point of view the best analysis is done by Aswath Damodaran in his book about investment valuation (2011).

Risk-free rate criteria

There are two criteria that a return rate of an asset can be considered as risk-free: 1) First the asset does not have any default risk. This excludes private entities, since even the largest and safest ones have some measure of default risk. The only securities that have a chance of being risk-free are government securities, not because governments are better than corporations, but because they usually control the printing of currency. 2) Secondly the asset must not have any reinvestment risk. The return rate has to be ensured fo the whole period of time.

Discounting Period

Government zero-coupon bonds and securities have different return rates for different time horizons. Usually the return rate increases with longer time horizons. Well-behaved term structures would include an upward-sloping yield curve, where long-term rates are at most 2 to 3 percent higher than short-term rates. For each maturity the investor gets a different guaranteed return on the investment. That means that each maturity has a specific discount rate.

Most DCF valuations use only one risk-free rate corresponding to a specific maturity. In most cases they take long term Treasury bonds, because cash flows mainly occur years away from present time $(t=0)$ of the valuation. But be aware that this is an approximation and can be false in some cases.

Currency

The risk-free rate used to come up with expected returns should be measured conisistently with how the cash flows are measured. Thus, if cash flows are estimated in nominal U.S. dollar terms, the risk-free rate will be the U.S. Treasury bond rate. This also implies that it is not where a firm is domiciled that determines the choice of a risk-free rate, but the currency in which the cash flows of the firm are estimated. If we assume purchasing power parity, then differences in interest rates reflect differences in expected inflation. Both the cash flows and the discount rate are affected by expected inflation; thus, a low discount rate arising from a low risk-free rate will be exactly offset by a decline in expected nominal growth rates for cash flows, and the value will remain unchanged. If the difference in interest rates across two currencies does not adequately reflect the difference in expected inflation in these currencies, the values obtained using the different currencies can be different.

Default Spreads

The interest rates on bonds are determined by the default risk that investors perceive in the issuer of the bonds. This default risk is often measured with a bond rating, and the interest rate that corresponds to the rating is estimated by adding a default spread to the riskless rate. An Euro government bond from Greek or Italian government has a higher default spread than a bond emitted by German government. You always have to subtract the default spread from the effective bond interest rate to get the risk-less (or better default-free) rate.

Conclusion

In most books concerning capital budgeting and DCF analysis the risk-free rate is the interest rate of a long term Treasury government bond. But also maturity, currency and default risk of the bond emitter have to be considered to get the right interest rate of a default-free asset. The investment analyst has to be aware of that and also has to know the inaccuracy when making approximations.

Investment Return Requirement

Joachim Kuczynski, 02 July 2022

In this post I want to give a derivation of the return requirement of an additional investment opportunity for an investor having an existing investment / security portfolio. In my point of view this is the key point of portfolio theory to understand the discounting of cash flows in a DCF analysis.

Let us assume an investor which owns a portfolio of investments or securities with relative shares $x_i$ having annual return rates $R_i$ and standard deviations of the annual return rates $\sigma\left( R_i \right)$ . The variance of the portfolio return rate is given by:

$var\left( R_P \right)=\sum_{i}^{}x_i\text{cov}\left( R_i, R_P \right) \text{, or}$

$var\left( R_P \right) =\sum_{i}^{}x_i\sigma\left( R_i \right)\sigma\left( R_P \right)\text{corr}\left( R_i, R_P \right)$

Dividing both sides by standard deviation $\sigma\left( R_P \right)$ gives the standard deviation $\sigma\left( R_P \right)$ :

$\sigma\left( R_P \right)=\sum_{i}^{}x_i\sigma\left( R_i \right)\text{corr}\left( R_i, R_P \right)$

That means that the incremental risk contribution of each investment to the risk of the portfolio ist $\sigma\left( R_i \right)\text{corr}\left( R_i, R_P \right)$ .

Instead of including a new investment into the portfolio the investor can also increase the return of the protfolio by increasing the risk of the portfolio. This reward-to-volatility ratio of the tangential portfolio is given by the Sharpe Ratio:

$\frac{E\left( R_P\right)-r_f}{\sigma \left( R_P \right)}$

$E\left( R_P\right)$ is the expected value of $R_P$ and $r_f$ is the risk-free or default-free rate. The investor wants to invest in the new opportunity, if the additional return rate of this investment is higher than an investment in the existing portfolio with the same risk changes. Hence we obtain the requirement to invest in the new investment opportunity:

$\text{E}\left( R_i \right)-r_f > \sigma\left( R_i \right)\text{corr}\left( R_i,R_P \right)\frac{E\left( R_P\right)-r_f}{\sigma\left( R_P \right)}$

With that we can define the sensitivity $\beta_i^P$ of the new investment to the existing portfolio:

$\beta_i^P=\frac{\sigma\left( R_i \right)\text{corr}\left( R_i,R_P \right)}{\sigma\left( R_P \right)}$

Substituting with $\beta_i^P$ the requirement for the new investment becomes the well-known equation:

$\text{E}\left( R_i \right) > r_f+\beta_i^P\left( E\left( R_P\right)-r_f \right)$

With that we can define a minimal annual return rate of the investment $r_i$ :

$r_i = r_f+\beta_i^P\left( E\left( R_P\right)-r_f \right)$

This is the right (leveraged) discount rate for cash flows financed by equity. It is the minimum rate at which an investor would decide to allocate the new investment opportunity in his portfolio, because the expected risk-adjusted return rate is higher that the risk-adjusted rate of the existing portfolio. It is easy to see that each cash flow has to be discounted with its specific risk-adjusted rate, when they have different risks. Because of the additivity of net present values the investor can discount each cash flow seperately and sum up the NPV of all cash flows. This is called component cash flow procedure, see this post.

If cash flows are financed by debt and equity, the discount rate is the weighted average of debt and equity return rate requirements (WACC).

Quite often the portfolio of a so called marginal investor is not known in detail. One possibility is to assume that his portfolio consists of all available securities in the market with its specific weighted shares. With the assumptions of the Capital Asset Pricing Model (CAPM) the efficient tangential portfolio is the market protfolio and the expected portfolio return rate is the expected return rate of the market. In most cases the S&P500 is taken as reference portfolio.

NPV Sign and NPV Timeline

In this post I want to discuss the sign of NPV when time scale is shifted by an amount of $\Delta t$ . The sign of NPV indicates whether an asset generates value to the fund providers (debt and equity) or not. If only the amount, but not the sign of NPV changes by a time shift, the decision to allocate the project or not does not change. That means that the decision itself does not depend on the time scale. In many books I have read that argument. But is this really true?

Project Cash Flow Procedure

The project cash flow procedure (PCFP) takes the same annual discount rate $r$ for all cash flows $C_i$ . By substituting $\alpha = ln (1+r)$ we can write $e^{- \alpha t}$ instead of $(1+r)^{-t}$ . The NPV of the project at the “present” time $t=0$ without time shift, let´s name it $NPV_0$ , is:

$NPV_0=\sum_{i}^{}E(C_i) e^{-\alpha t_i}$

$E(C_i)$ is the expected value of the i-th cash flow component. When we shift time by $\Delta t$ , we get a new NPV, let`s call it $NPV_ {\Delta t}$ :

$NPV_ {\Delta t} =\sum_{i}^{}E(C_i) e^{-\alpha (t_i+ {\Delta t} )}$

$NPV_ {\Delta t} = e^{ -\alpha \Delta t} \sum_{i}^{}E(C_i) e^{-\alpha (t_i )}= e^{ -\alpha \Delta t} NPV_0$

Because $e^{ -\alpha \Delta t}>0$ , the sign of $NPV_0$ and $NPV_ {\Delta t}$ is the same for all $\Delta t$ . That means the decision, when based on the NPV sign, remains the same: Invest in case of positive NPV and do not invest in case of negative NPV. In the PCFP a time shift does not affect the decision.

Component Cash Flow Procedure

The component cash flow procedure (CCFP) takes the specific appropriate risk adjusted discount rate for each cash flow. That means that you have a specific discount rate $r_i$ , or $\alpha _ i = ln (1+r_i)$ respectively, for cash flow $C_i$ . The NPV of the project without time shift, $NPV_0$ , is:

$NPV_0=\sum_{i}^{}E(C_i) e^{-\alpha_i t_i}$

When we shift time by $\Delta t$ , we get a new $NPV_ {\Delta t}$ :

$NPV_ {\Delta t} =\sum_{i}^{}E(C_i) e^{-\alpha_i (t_i+ {\Delta t} )}$

We cannot make further simplifications because each term has an individual discount rate $\alpha_i$ . That means that the sign of NPV with time shift does not have to be the same as the NPV without time shift, the sign can change. Hence the decision whether to allocate the project or investment can also change. Furthermore that means that we have to pay attention to take the right time scale in the calculation to come to the right decision.

Conclusion

In this post I described the advantages of the CCFP over the PCFP. The PCFP is only a simplification of the CCFP, PCFP can lead to wrong decisions. Because CCFP is the preferred and valid procedure of calculation, we can state that the sign of NPV can change by a time shift in general. The decision based on the NPV sign is only valid for the “present” time, which is $t=0$ in the DCF calculation. If you want the calculation to be the basis for an investment decision, you have to ensure that $t=0$ in the calculation is the point in time of the decision!

Several companies set $t=0$ at the beginning of the project`s revenues or the first investment cash flows. But this neglects the uncertainty and riskiness of cash flows from the decision point of time to their starting points. That is false in general, because of the arguments above. $t=0$ has to be the point in time of the decision. Otherwise you can generate false corporate decisions.

Incremental Free Cash Flows

In this post I want to point out some important characteristics of incremental free cash flows in a discounted cash flow (DCF) analysis. Incremental cash flows after taxes are the basis of each DCF analysis and in this way of each investment valuation.

Relative View to Alternative Scenario

Incremental means that the cash flows are caused by a positive investment decision. Positive decision means that the investment project is decided to be realized. Incremental cash flows are the cash flows that are additional to the cash flows of a negative investment decision (zero scenario or better alternative scenario). But to get the additional cash flows to the alternative scenario, you have to know the alternative scenario! In many cases, especially in “green field” projects, it is easy. There are simply no cash flows concerning your company in the alternative scenario. But it can become more complicated, if the alternative scenario depends on other investment project decisions that are not decided yet. In this case the investment projects are interrelated. Investments projects can for example require the same investment resources. But the company has to purchase it only once. If resources are used by several investment projects, the investment planning and DCF calculation must be done in a comprehensive company view. Only in this way you can allocate the investments to the investment projects. But nevertheless this is not always clear, because this allocation to a certain project can be ambiguous. Many companies have a central investment planning department which ensures that interrelated investment projects are harmonized.

A company can have the choice between two exclusive scenarios, which are both unprofitable stand-alone. But the company has to choose on of these two alternatives. When making a DCF analysis of one of these scenarios you have to take into account that the other scenario is not zero cash flow but the second excluding alternative scenario. That can lead to the fact that the DCF analysis becomes profitable because the negative effects of the alternative scenario can be prevented. Can that be true? Yes, because a DCF analysis is always decision focused and the decision can be more profitable than the realization of the alternative scenario. It is very important to have in mind this relative characteristic of a DCF analysis.

Sunk Costs and Opportunity Costs

An additional crucial point is that the incremental free cash flows must be caused by the decision to realize an investment project (principle of cause and effect). Costs that are linked to the project but cannot be influenced by the investment decision itself are so called sunk costs. Sunk costs are not part of the incremental free cash flows. In this way your DCF calculation shows whether the decision to realize the investment project is profitable for the company or not. But generally it does not show whether the project itself is profitable or not. Management cannot avoid sunk costs, hence the calculation show straightforward the ability to influence the future development. But you also have to take into account opportunity costs. These are negative cash flows effects caused by the investment decision but actually part of other projects. For example, the launch of a new product can substitute the volume of another (just released) investment project. Another example is a price reduction on the product which volume is part of the contribution cash flows of another investment project. This relationship also has to be considered if you are doing a recalculation of an investment project.

Conclusion

You should always consider these two point in a DCF analysis: 1) The relative view compared to the alternative scenario and 2) the decision making focus ignoring sunk costs and including opportunity costs. Sometimes companies are making recalculations of their investment projects. Recalculations can only be compared with the original, decision focused DCF analysis when taking the same assumptions. In an interrelated investment world you cannot recalculate one investment project without knowing the relationship to the other investment projects.

WACC with Tax Shield

In this post I want to provide a derivation of the discount rate that includes savings because of interest tax shield. Further I can show a general expression for tax shields implementation, wherein this well known WACC formula is only a special case:

$WACC=\frac{D}{D+E}r_{D}\left( 1-t \right)+\frac{E}{D+E}r_{E}$

The formula includes “ $-t$ ” that comes from tax shield savings. $D$ and $E$ stand for debt and equity of the firm, $r_D$ and $r_E$ are the required return rates for debt and equity, $t$ is the marginal tax rate.

General case

We consider one time period starting at $t_0$ and ending at time $t_1$ . In $t_1$ we have a cash flow excluding tax shield of $C_1$ and an absolute tax shield value of $T_1$ . $r$ is the discount rate without tax shield. We are searching a discount rate $r^*$ that allows us to discount the cash flow excluding tax shield but including the tax shield effect in the present value in $t_0$ . Hence we have to adapt the discount rate. The discounted value of the cash flows in $t_0$ has to be the same for both discount rates:

$\frac{C_1+T_1}{1+r}=\frac{C_1}{1+r^{*}}$

That leads to a general relationship of $r^{*}$ and $r$ :

$r^{*}=\frac{C_1r-T_1}{C_1+T_1}$

That expression allows us to include a cash flow $T_1$ into the discount rate and to discount the cash flows in $t_1$ excluding $T_1$ with the adapted discount rate $r^*$ . It enables us to calculate the discount rate $r^*$ from the cash flows $C_1$ and $T_1$ in $t_1$ . You do not require any values from $t_0$ . Especially there is no need to have knowledge about the capital structure of the company. But for sure the capital structure is required to calculate $T_1$ in most cases.

Including capital structure

Next we want to consider the capital structure in $t_0$ . The discounted asset value consists of debt $D_0$ and equity $E_0$ . The discounted value of the cash flows must be the sum of debt and equity. With $\left( D_0 + E_0 \right) \left( 1+r \right) = C_1 + T_1$ we obtain:

$r^*=r-\frac{T_1}{D_0+E_0}$

Setting $r$ as weighted average return rates of debt and equity without tax shield we get:

$r^*=\frac{D_0}{D_0+E_0}r_{D}+\frac{E_0}{D_0+E_0}r_{E} -\frac{T_1}{D_0+E_0}$

Famous WACC after taxes

In most cases the tax shield is the interests paid on $D_0$ times the marginal tax rate $t$ . That means $T_1=D_0 r_D t$ . Hence we get the well known expression for $r^*$ :

$r^*=\frac{D_0}{D_0+E_0}r_{D}\left( 1-t \right)+\frac{E_0}{D_0+E_0}r_{E}$

This is the discount rate or “WACC” after taxes which is quoted in most books. But take care! It is only valid, if really the complete amount of interests paid can be deducted from taxes. Sometimes the company does not have enough profit to deduct all interest payments. In other cases the amount of tax deduction is limited by some constraints. In these two cases the previous formula does not work any more. The equation also shows that the capital structure in $t_0$ is important and not the capital structure in $t_1$ .

Maximum constraint of tax shield

If the company has for example a maximum for the tax shield $T_1^{\text{max}}$ , maybe a maximum share of EBITDA in $t_1$ , we obtain another expression:

$r^*=\frac{D_0}{D_0+E_0}r_{D}+\frac{E_0}{D_0+E_0}r_{E} -\frac{ \min \left( D_0 r_D t , T_1^{\text{max}} \right)}{D_0+E_0}$

Or if the company has tax shield savings from other periods in $t_1$ , the equation is not valid, too.

In my point of view, the Adjusted Present Value (APV) approach is much better than the WACC approach for the implementation of tax shield. Each time period has clear tax shield amounts. And in complex cases you do not have to adapt the WACC in each time period.

Component Cash Flow Analysis

Project and component cash flow procedure

In this post I want to give some remarks on the component cash flow procedure. I am using this approach in my DCF analysis. It is the only correct way to discount the cash flows of complex investments, projects or diversified firms. In investment valuation many analysts take the WACC of the company, the business unit or the investment project to discount the cash flows. Taking only one single discount rate in a valuation is called project cash flow procedure (PCFP)

But most projects consist of a mixture of cash flows with different risks. To take this into account the present value of each cash flow should be calculated using a discount rate appropriate for its risk. The present values of all of the cash flow components should then be summed up. Projects should hence be selected using the present value criterion applied to its total present values. This approach is called component cash flow procedure (CCFP).

Disadvantages of using a single discount rate

In general, it is possible to find a composite discount rate for a project that gives the same NPV for the project as the NPV derived from the component cash flow procedure. The net cash flows can be determined that gives the same present value as the sum of the present values of its components. But there are at least three problems in applying one discount rate to the sum of the cash flows (Bierman, Smidt / Advanced Capital Budgeting, 2007).

Any change in assumptions about the project will tend to lead to a change in the composite discount rate. Changing the life of the project or the proportion of any of its cash flow components would likely require a different composite discount rate for the total cash flows.
If the correct composite discount rate is applied to the net cash flows of a project, then although the NPV of the project will be correct, the present values assigned to the cash flow components using this rate will be inaccurate. For example, the present value of the depreciation tax shields will usually be underestimated. In addition, the present value of the total cash flows in a particular year or a particular period will usually be inaccurate. This may lead to errors in decisions, such as estimating the value of the project at various future dates.
If the cash flow mixture is changed, the present value calculated using the previous composite discount rate would not produce accurate present values. This is particularly important in making choice between mutually exclusive alternatives that frequently involve a change in the mixture of cash flows, for example, the substitution of capital for labor.

If the project life is finite and greater than one year, then finding the composite discount rate of a project requires finding an IRR. There may be projects for which an IRR does not exist, or is not unique. For those projects, there may be no composite rate, or the composite rate may not be unique.

My proposal for appropriate discount rates

I propose the following way to discount the cash flows in a DCF analysis:

Market related (contribution) cash flows that depend on the overall market development should be discounted with their appropriate risk adjusted WACC, corrected by extracting Operating Leverage. For this I take the CAPM (including additional country risk premiums for non-diversifiable country specific risks) and the APT. These WACC should include appropriate default free interest rates, market and country risk premiums, the appropriate debt to equity ratios, equity betas and marginal tax rates. Revenues, variable costs, taxes and expenses for working capital are typically part of these market contribution cash flows. If you have a project with revenues in various markets with different currencies and risks, you have to discount each cash flow with its appropriate discount rate.
Capex / investment cash flows should be discounted with the reinvestment rate of the corresponding currency.
One time payments and expenses should be discounted with the risk-free rate of the corresponding currency.
Fixed costs should be discounted with the reinvestment rate of the corresponding currency. In some real options, e.g. switch options, savings in fixed costs are part of the option value. Option values are discounted with the risk-free rate, which is close to the WACC with a CAPM beta of 0. In this way DCF and ROA concepts match.
Leasing revenues and expenses should be discounted with the risk-free rate of the corresponding currency. The buy versus lease decision illustrates well the desirability of using different discount rates for cash flows with different characteristics. The use of different discount rates for different cash flow components is a widely accepted practice in analyzing buy vs lease problems.
Interests and tax shield savings depend on the leverage strategy of the company. If the amount of debt is fixed over time, the discount rate should be the risk-free rate of the corresponding currency. If the share of debt is kept constant over time, the discount rate should be the specific risk-adjusted market WACC.

WACC, Return Rates & Betas with Debt

Joachim Kuczynski, 02 April 2021

In this post I want to summarize some interesting results concerning equity return rates , betas and WACC of a levered company. Regarding the market value balance sheet of a firm we can state that the value of the unlevered firm VU plus the present value of the tax shield VTS must be the same as the sum of levered equity E and debt D:

$VU+VTS=E+D$

Further the rates of return on each side of the balance sheet are the weighted average of the component rates of return:

$r_A\frac{VU}{VU+VTS}+r_{TS}\frac{VTS}{VU+VTS}=r_E\frac{E}{E+D}+r_D\frac{D}{E+D}$

Substituting VU in the rate of return expression we get a general form of the equity return rate:

$r_E=r_A+\left(r_A-r_D\right)\frac{D}{E}-\left(r_A-r_{TS}\right)\frac{VTS}{E}$

Consequently the general form of CAPM beta is given by:

$\beta_E=\beta_A+\left(\beta_A-\beta_D\right)\frac{D}{E}-\left(\beta_A-\beta_{TS}\right)\frac{VTS}{E}$

The WACC is defined as the weighted average of equity and debt return rates including tax shield at corporate income tax rate $T_C$ . If the tax shield savings are proportional to the taxes paid (see WACC with Tax Shield), the WACC is given by:

$WACC=r_E\frac{E}{E+D}+r_D\left(1-T_C\right)\frac{D}{E+D}$

Substituting the equity return rate we get a general form of the WACC:

$WACC=r_A\left(1-\frac{VTS}{V}\right)-r_DT_C\frac{D}{V}+r_{TS}\frac{VTS}{V}$

$r_A$ , $r_D$ , $r_{TS}$ are the return rates of the unlevered asset, debt and tax shield. V and VTS are the values of the levered firm and the tax shield. D is the amount of debt, V is the value of the levered firm, namely the sum of equity and debt.

Modigliani and Miller: Constant debt value
If the firm keeps its dept value D constant, there are no specific market risks concerning the tax shield. Therefore we can set the tax shield discount rate $r_{TS}$ equal to the debt discount rate, $r_D$ . The tax shield present value with constant debt D is:

$VTS=\sum_{j=1}^{\infty}{DT_C\left(\frac{1}{1+r_D}\right)}^j=\frac{DT_C}{r_D}$

Hence we get simplified expressions for equity return, equity beta and WACC:

$r_E=r_A+\left(r_A-r_D\right)\frac{D}{E}\left(1-T_C\right)$

$\beta_E=\beta_A+\left(\beta_A-\beta_D\right)\frac{D}{E}\left(1-T_C\right)$

$WACC=r_A\left(1-T_C\frac{D}{E+D}\right)$

Assuming that debt interest rate does not depend on the market return rate (CAPM) we can set $\beta_D=0$ . Hence we get the well-known Hamada equation for levered beta:

$\beta_E=\beta_A\left(1+\frac{D}{E}\left(1-T_C\right)\right)$

It is important to realize that Hamada’s equation is only valid if the value of debt is kept constant over time.

Harris and Pringle: Constant leverage ratio
Constant leverage ratio means that debt value is proportional to the value of the unlevered firm. According to Harris and Pringle that results in $r_{TS}=r_A$ .

$r_E=r_A+\left(r_A-r_D\right)\frac{D}{E}$

$\beta_E=\beta_A+\left(\beta_A-\beta_D\right)\frac{D}{E}$

$WACC=r_A-r_DT_C\frac{D}{E+D}$

But we have to take care. Miles and Ezzell, Arzac and Glosten have shown that you have a tax shield discount rate of $r_D$ in the first period, and of $r_A$ in the following periods to have a constant leverage ratio over time. The premise of $r_{TS}=r_A$ does not hold.

Miles and Ezzell
With a perpetuity growing rate g of debt and discounting in the first period with $r_D$ instead of $r_A$ we obtain:

${VTS}^{ME}=\frac{Dr_DT_C\left(1+r_A\right)}{\left(r_A-g\right)\left(1+r_D\right)}$

Harris and Pringle
Taking the formula of Miles and Ezzell and setting $r_TS$ equal to $r_A$ in the first period, we get the a simplified expression for $VTS$ :

${VTS}^{HP}=\frac{Dr_DT_C}{\left(r_A-g\right)}$

General debt ratio
If the amount of leverage is flexible and not constant or growing with a constant growth rate over time, the previous formulas do not work. In this case you have to use the APV method, in which you calculate the tax shield in each time period seperately.

Baldwin Rate of Return | MIRR

Joachim Kuczynski, 05 March 2021

Baldwin rate definition

The modified internal rate of return (MIRR), or Baldwin rate of return respectively, is an advancement of the internal rate of return (IRR). But also the MIRR can be misleading and can generate false investment decisions. The MIRR is defined as:

$MIRR=\sqrt[n]{\frac{\text{FV(contribution cash flows,WACC)}}{\text{PV(invest cash flows, financing rate)}}}-1$

FV means the final value at the last considered period, PV stands for the present value.

Pitfalls of the Badwin rate

These points have to be considered carefully when applying the MIRR:

Cash flows in different countries, with different currencies, equity betas, tax rates, capital structure, etc. should be evaluated with specific risk-adjusted discount rates. In MIRR the project is profitable, if the rate of return is higher than the required WACC. But which WACC do we mean in projects with various differing cash flows ? No diversification of cash flows can be taken into account in MIRR. But that is crucial in evaluating international projects.
You need premises about the reinvestment rate of the contribution cash flows that affect the profitability of the project. These premises are not required in the (e)NPV concept. Hence you add an additional element of uncertainty in your calculation when using the MIRR, without any need.
Reinvesting contribution cash flows (numerator of the root) with the risk adjusted WACC means that the return of the project increases when the project risks and WACC increases. That cannot be true. In pinciple you should not use key figures that require assumptions about reinvestment return rates. You are evaluating a certain project and not of other unknown investment sources. In general you can discount all cash flows with its appropriate discount rate and capitalize it to the last period.
Does capitalizing (or rediscounting) a cash flow with a risk-adjusted discount rate to a future period make sense in general? I do not think so. To rediscount cash flows with a risk adjusted discount rate including a risk premium means that you are increasing the risk of the project. The project does not remain the same, because its risk increases. Only taking a riskless discount rate for reinvestments would not increase the risk of the project. The MIRR comes from a classical perspective with no risk adjustment of the discount rates. If you are using risk-adjusted discount rates, you are mixing two concepts that do not fit.
An additional positive cash flow must improve the profitability of the project. If you add an additional, small cash flow in an additional period $n+1$ , the Baldwin rate can decrease. This is because the number of periods increases and the value of the root decreases. Cases that lead to wrong results are not acceptable for decision key figure.
If you have e.g. an after sales market with small positive cash flows, the Baldwin rate decreases by considering these cash flows in your calculation. This is because the number of periods increases and the $n$ -th root decreases. Thus an after sales market cannot be implemented in your calculation.
You have to define clearly, which cash flow is in the numerator and which is in the denominator of the root. There is no clear and logic distinction. Thus you can find different definitions in literature. Anyway avoid to take balance sheet definitions of “investment”. Note that besides investments also fixed costs and leasing payments have to be discounted with a default free discount rate in general.
You cannot compare mutual exclusive investment projects, if the investments or the project periods are different.
You can also not evaluate investment projects with negative value contribution to the firm. But anyway such projects exist and have to be decided.
All cash flows should be considered as expected value of a probability distributions. The expected value of the Baldwin rate is not the Baldwin rate of the expected values of the cash flows.

Conclusion

The (e)NPV concept is much better than the MIRR or Baldwin Rate of Return. The (e)NPV does not have all the pitfalls mentioned above. Further you can also evaluate and compare value-loosing investment alternatives and do not need any premises about reinvestment rates. There are only disadvantage of the MIRR / Baldwin rate compared to the (e)NPV, try to avoid the application of MIRR / Baldwin rate.

Operating Leverage

Operating leverage is the sensitivity of an asset’s value on the market development caused by the operational cost structure, fixed and variable costs. The asset can be a company, a project or another economic unit. A production facility with high fixed costs is said to have high operating leverage. High operating leverage means a high asset beta caused by high fixed costs. The cash flows of an asset mainly consists of revenues, fixed and variable expenses:

cash flow = revenues – fixed expenses – variable expenses

Costs are variable if they depend on the output rate. Fixed costs do not depend on the output rate. The (present) value of the asset, $V_{asset}$ , is the sum of its cash flows’ (present) values, namely revenue $V_{rev}$ , variable costs $V_{var}$ and fixed costs, $V_{fix}$ . Present values are linear, for the value of the asset we can write:

$V_{asset} = V_{rev} + V_{fix} + V_{var}$

Rearranging leads us to:

$V_{rev} = V_{asset} - V_{var} - V_{fix}$

Those who receive the fixed expenses are like debtholders in the project. They get fixed payments. Those who receive the net cash flows of the asset are like shareholders. They get whatever is left after payment of the fixed expenses. Now we analyze how the beta of the asset is related to the betas of revenues and expenses. The beta of the revenues is a weighted average of the betas of its component parts:

$\beta_{rev}=\beta_{asset}\frac{V_{asset}}{V_{rev}}-\beta_{var}\frac{V_{var}}{V_{rev}}-\beta_{fix}\frac{V_{fix}}{V_{rev}}$

The fixed expense beta is close to zero, because the fixed expenses do not depend on the market development. The receivers of the fixed expenses get a fixed stream of cash flows however the market develops. That means $\beta_{fix} = 0$ . The betas of revenues and variable expenses are more or less the same, because they are both related to the output. Therefore we can substitute $\beta_{rev}$ for $\beta_{var}$ .

$\beta_{asset}=\beta_{rev}\frac{V_{rev}+V_{var}}{V_{asset}}$

Setting $V_{rev} + V_{var} = V_{asset} - V_{fix}$ we obtain:

$\beta_{asset}=\beta_{rev} \left( 1 - \frac{V_{fix}}{V_{asset}} \right)$

This is the relationship of asset beta to the beta of turnover. The asset beta increases with increasing fixed costs. As an accounting measure we define the degree of operating leverage (DOL) as:

$\text{DOL}= 1 + \frac{\left| \text{fixed exp.} \right| }{\text{profits}}$

The degree of operating leverage measures the change in profits when revenues change.

Valuing the equity beta is a standard issue in DCF analysis. In many cases you take an industry segment beta and adjust it to your company or project. The adjustment of the industry beta also includes the adjustment of operating leverage. We assume that $\beta_{\text{revenue}}$ is the same for all companies in the industry segment. $\beta_{\text{revenue}}$ is the beta of the segment without operating leverage. The $\beta_{\text{asset}}^{\text{ind. segm.}}$ is the average asset beta of the industry segment, which has an average ratio of fixed expenses to profits. $\beta_{\text{asset}}^{\text{ind. segm.}}$ is provided by public databases.

For detailed information see: Brealey/Myers/Allen: Principles of Corporate Finance, 13th edition, p. 238, McGraw Hill Education, 2020)

After-Tax Discount Rate

In this post I want do derive the after-tax discount rate from the before-tax discount rate. “Before tax” means that the tax shield is not considered in the discount rate. It does not mean that the tax expenses (without tax shield) are not considered in the free cash flow. The tax expenses (without tax shield) are a part of the free cash flow in the before-tax and in the after-tax discount rate. For further information have a look at my other post WACC with Tax Shield. Abbreviations:

$r$ … before-tax discount rate
$r^{*}$ … after-tax discount rate
$L$ … rate of debt to sum of equity $E$ and debt $D$ , $L=D/(E+D)$
$r_D$ … debt interest rate
$r_E$ … equity interest rate
$t$ … marginal corporate tax rate

We assume that the values of $r_D$ , $r_E$ and $L$ are known. Then the before-tax discount rate is:

$r=\left( 1-L \right)r_{E}+Lr_D$

Rearranging the above to solve for $r_{E}$ we have:

$r_{E}=\frac{r-Lr_D}{1-L}$

The after-tax discount rate at a constant leverage rate is:

$r^{*}=\left( 1-L \right)r_{E}+L\left( 1-t \right)r_D$

This is the famous equation most financial analysts might know. The factor “-t” comes from the tax shield and decreases the discount rate. Hence the discount rate after taxes is lower than the return rate before taxes. But you have to take care. This after-tax formula is only valid if the leverage rate $L$ remains constant. Additionally it assumes that the total amount of tax expenses can be deducted by tax shield. If these two premises are not true, the previous formula does not work and you have to an analyze the topic with the adjusted present value (APV) approach. For a general view see this post. By substituting $r_{E}$ we get:

$r^{*}= r -Ltr_D$

This formula can be useful, because you do not have to know the equity return rate to calculate the after-tax return rate. But have in mind that this is only valid, if the leverage ratio is constant and the total tax shield amount can really be deducted from the tax expenses.

Pitfalls of Discounted Cash Flow Analysis

Correctly appraising capital projects with DCF analysis methods requires knowledge, practice and acute awareness of potentially serious pitfalls. I want to point out some important errors in project appraisal and suggest ways to avoid them. For many people DCF analysis seems to be quite easy, but it can be very difficult for complex projects. Here are some crucial issues from my point of view:

Decision focus: The calculation is focused on making the right decision concerning a project or an investment. That can be different from a calculation including all expenditures of the project or investment, e.g. sunk costs. For further comments concering this topic see Incremental Free Cash Flows.
Point of view: It has to be defined clearly from which perspective you are doing the decision and calculation. For example, the calculation can be different from the view of a business area and from the view of the overall company. The right perspective to the decision problem determines the relevant incremental cash flows.
Investment: Define clearly what you mean when talking about “investment”. Avoid the balance sheet view, look at investment as initial expenditures required for later contribution cash flows. In my point of view the term “investment” is best defined as commitments of resources made in the hope of realizing benefits that are expected to occur over a reasonably long period of time in the future.
Cash flows: A clear view of cash flow is important, avoid views from accounting and cost accounting, e.g. depreciation. And take into account tax effects.
Incremental cash flows: The correct definition of incremental cash flow is crucial. It is the difference between the relevant expected after-tax cash flows associated with two mutually exclusive scenarios: (1) the project goes ahead, and (2) the project does not go ahead (zero scenario). Sunk costs must not considered. For further comments see Incremental Free Cash Flows.
Comparing scenarios: Alway be aware of having a relative sight between the cash flow scenarios. Sometimes it is not so easy to define what would happen in the future without the project (zero scenario).
Risk-adjusted discount rates: Risk adjustment of discount rates has to be done for all (!) cash flows of the investment project that have significant risk differences: Fixed costs, investment expenses, one time expenses and payments, expenses for working capital, leasing, tax shields and contribution cash flows (turnover and variable costs) in various markets. For more infos concerning risk adjusted discount rates see Component Cash Flow Procedure.
Key figures: The only key figure that is valid for all types of projects and investment decision is the famous NPV. All other well-known figures like IRR, Baldwin rate, … are leading to false decisions in some cases. NPV also allows to build the bridge to financial calculation approaches like option valuation. Payback and liquidity requirements have to be considered carefully additionally to NPV.
Expected versus most likely cash flows: Quite often analysts take most likely cash flows. The right way is to consider the expected value (mathematical definition) of the cash flows.
Limited capacity: Do not forget internal capacity limitation when regarding market figures. Limited capacity has also to be considered when constructing the event tree in real options analysis. Besides that the temporal project value development with contribution cash flow’s discount rate has to be ensured in the binomial tree.
Hurlde rates: Avoid hurlde rates for project decisions, because the can also lead to false decisions. Especially when you take one hurdle rate for different projects.
Cash flow forecasting: Forecasts are often untruthful. Try to verify and countermeasure cash flows from different sources.
Inflation: Be careful considering inflation. In multinational project it might influence the foreign currency location’s required return. You can also consider a relationship between inflation rate and expected future exchange rates according to the purchasing power parity (PPP).
Real and nominal discount rates and cash flows: The procedure should be consistent for cash flows and discount rates. Usually we take nominal values for the calculation.
Real options: A DCF analysis should always be linked to a real options analysis. The more flexibility is in the project the more important is a real options analysis. Risk adds value to real options.
Precise cash flow timing: The influence of timing intervals can be significant. You can choose smaller time intervals in crucial time periods to increase accuracy.